Biography
Biography: Vladimir I Andreev
Abstract
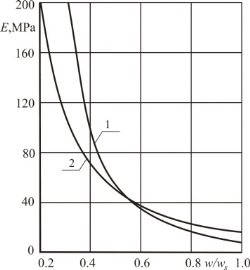
The rupture of water and sewer pipes leads to numerous accidents (subsidence, swelling, etc.). This is especially characteristic for clay, loess, peat and other soils. We consider the stress state of clay array at different models on fluid propagation from a fracture site. Accordingly, the problems are solved in cylindrical and spherical coordinates. The problem is solved by the methods of stationary and non-stationary moisture elasticity. The feature of the calculation is the accounting of the inhomogeneity of the clay during moistening. Figure 1 shows the dependence of the clay deformation modulus on moisture. Depending on the depth of the pipe where the fracture occurred, the ground pressure can be considered axisymmetric (large depth) and non-axisymmetric (small depth). Accordingly, one-dimensional and two-dimensional problems of moisture elasticity are considered. Analytical and numerical-analytical solutions are obtained. An analysis is made of the convergence of the Fourier series as a function of the number of terms in the series. As a result of calculations, it was found that taking into account the inhomogeneity caused by the change in the deformation properties of bodies leads to a significant change in the stress state of bodies compared to the calculation of homogeneous bodies. In clay soils, against the background of a marked reduction in the peaks of compressive stresses, the most dangerous maximum tensile stresses for a cylindrical model increase by 53%, and for a spherical stress increase by 38%.